The average score of a cricketer for 13 matches is 42 runs. If his average score for the first 5 matches is 54, then what was his average score (in runs) for last 8 matches?
37
39
34.5
33.5
Answer : Option C.
Total Score = Average * Number of matches
Total score of 13 matches = 13 × 42 = 546
Total score of first 5 matches = 5 × 54 = 270
Therefore, total score of last 8 matches = 546 - 270 = 276
Average = 276/8 = 34.5
Hence the answer is option C
If 1/a - 1/b = 1/a-b, then the value of a3+b3is
0
-1
1
2
Answer : Option A.
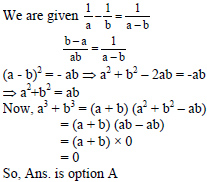
If a2+b2+c2 = ab+bc+ca , then (a+c)/b is equal to
1
2
3
4
Answer : Option B.
If the graphs of the equations x + y = 0 and 5y + 7x = 24 intersect at (m, n), then the value of m +n is
2
1
0
-1
Answer : Option C.
By solving the given two equations, we get the intersection point (12, - 12).
So, m = 12, n = -12
Hence, m + n = 12 – 12 = 0 So, ans. is option C.
A function f(x) is defined as f(x) = f(x – 2) - x(x + 2) for all the integer values of x and f(1) + f(4) = 0. What is the value of f(1) + f(2) + f(3) + f(4) + f(5) + f(6)?
0
89
-89
None of these
Answer : Option C.
Let S = f(1) + f(2) + f(3) + f(4) + f(5) + f(6)
As f(1) + f(4) = 0, therefore S = f(2) + f(3) + f(5) + f(6) ------ (1)
f(2) = f(0) - 8
f(3) = f(1) - 15
f(4) = f(2) - 24 = f(0) - 32
f(5) = f(3) - 35 = f(1) - 50
f(6) = f(4) - 48 = f(0) - 80
Put the above values in equation (1), we get
S = f(0) - 8 + f(1) - 15 + f(1) - 50 + f(0) - 80
S = 2(f(0) + f(1)) - 153 ------ (2)
As we already know f(1) + f(4) = 0 ⇒f(1) + f(0) - 32 = 0 ⇒f(1) + f(0) = 32
Putting this value in equation 2, we get S = 2(32) - 153 = -89
So, Ans is option C.
What annual payment will discharge a debt of Rs. 6,450 due in 4 years at 5% per annum simple interest?
1400
1500
1550
1600
None of these
Answer : Option B.
Let the annual installment be rs. x
therefore (x + x*3*5/100)+(x + x*2*5/100)
+(x + x*1*5/100)+x =6450
=>115x/100+110x/100+105x/100+x=6450
=>115x+110x+105x+100x=6450*100
=>430x=6450*100
x=6450*100/430=rs.1500
The average of the first 100 positive integers is
100
51
50.5
49.5
49
Answer : Option C.
n(n+1)/2
1+2+3+....+n
therefore average of these numbers=n+1/2
therefore required average
100+1/2=50.5
In a family, the average age of a father and a mother is 35 years. The average age of the father, mother and their only son is 27 years. What is the age of the son?
12 years
11 years
10.5 years
10 years
None of these
Answer : Option B.
Father+Mother=2*35=70 years
Father+Mother+Son=27*3=81 years
therefore Son's age=81-70=11 years
DIRECTIONS for the question 9 and 10 : Solve the following question and mark the most appropriate option
The length and breadth of a rectangle are increased by 20% and 40% respectively. What is the percentage increase in its area?
60%
68%
78%
Data inadequate
Answer : Option B.
Apply the percentage formula. The percentage increase in the area will be P + Q + PQ / 100. So we get the answer as 20 +
40 + 20 × 40/100 = 68%. i.e. 2nd option.
The diameter of a circle is 21 metres. It will take how many revolutions to cover a distance of 6.6 km?
80
50
200
100*
Answer : Option D.
No. of revolutions = Distance/circumference.
Distance = 6.6 × 1000 = 6600 metres.
Circumference = 2 × 22/7 × 21/2 = 66 metres.
No. of revolutions = 6600/66 = 100 revolutions. i.e. 4thoption